The uncertainty principle or the inequalities of indeterminacy

26 Avr 2019 by René Lebon

“Metiri est mentiri”

(Measurements lie)

Giordano Bruno

“Errare humanum est, persevare diabolicum”

Werner Heisenberg was born in Germany shortly after Planck’s discovery was made in 1900. This consisted of noting that energy exchanges could only occur via packets which were later called “photons. These packets of energy vibrate various ways at a certain frequency which, when multiplied by the universal constant for which the symbol is h, gives them this energy. This discontinuity brought about a revolutionary change in physics as a whole.

When he was around 20 years old, he learned Niels Bohr’s concept of the hydrogen atom, represented as a planetary system, with the electron spinning at various levels around the proton. These levels are a function of the constant h.

Suffering from a major pollen allergy, Heisenberg sought refuge on the island of Heligoland, north of Hamburg, where there are not many plants. There, he experienced a night when his light bulb moment occurred. For him, Bohr’s atom was just a representation in the image of the way the planets rotate around the Sun. In fact, this is estimated based on numerical measurements. He also came to have the idea of grouping these numbers together in a table where the lines and columns represent the energy and frequency values of the various spectral rays. Balmer had empirically found a mathematical formula that summarised all this.

So Heisenberg put together two tables; one concerning frequencies and one concerning amplitudes or, in other words, energy.

As an example, here is the table for frequencies f :

On a line, you place the various frequencies in order to go from level 1 to levels 1-2, 1-3,…, 1-n and, in a column, you place the frequencies 2-1,…, p-1. Adopting the same approach, you compile a table for the amplitudes a.

By applying multiplication rules to these two tables, you obtain the following example:

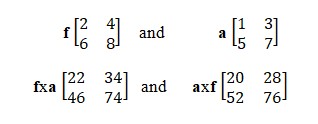
Note that the multiplication is not commutative; in other words f x a is different from a x f.

Upon returning to the University of Göttingen, Heisenberg talked about his discovery to his colleagues Born and Jordan. Born recognised the matrix calculation developed by Cayley in 1858. Won over by the idea of just considering the numbers to be the outcomes of experiments, they came up with the idea of establishing a relationship through a table concerning the positions q of particles with a movement quantity mv or p. They came to note that the difference between qp and pq is not nil or, in other words, these two magnitudes are not commutative.

So this observation then had to be put into a formula. Firstly, you have to specify that q and p are dependent simply for the following reasons: p is a pulse, or in other words mv, and is therefore a function of the particle’s velocity. However, you can only define a velocity through the variation in position q and v is defined via infinite approximation of two positions with an infinitesimal difference. This is the classic calculation of the derivative. The velocity can only be evaluated if you can assess the change in position in terms of space and time. Let’s refer to Appendix 4, where we can carry out a calculation, given a function Ψ(x), its variable x, and the derivative Ψ’(x). It is shown that the difference between x xΨ’(x) and Ψ’(x)x x is not nil. You obtain 1 or -1 depending on the order of the operations or, in other words, you obtain the same function Ψ(x) or its anti-symmetric value – Ψ(x). This is called “non-commutativeness”.

Let’s consider the value x (which is one-dimensional) for q (the position), with p being for the movement quantity mv.

The wave equation is Ψ = exp ipx/ħ

and its derivative is i/ħ exp ipx/ħ, for which the symbol is D. The operator of the wave function is Ψ(xD – Dx) or the inverse.

Depending on the order x, D, you obtain Ψ or its inverse – Ψ.

Let’s multiply the coefficient of D: i/ħ by iħ, simply changing its sign. The same applies for Ψ which is then multiplied by iħ. D may be considered to be a function of p.

This gives us xp – px = iħ. This operator is therefore not commutative.

Two remarks:

1) The exponential function exp x has the “unique” particularity of being both its derivative and its integral right down to the level of a constant. This means that the derivative varies in the same way as expx. This avoids the classical derivative problem, which is the value that the function trends to when its variation trends to 0.

And yet 0 is not accessible in quantum physics. The minimum action, namely Planck’s constant h, although extremely weak, is never nil. We will see below that h is indeed the cause of the indeterminacy relations. The whole of quantum mechanics was created from Planck’s observation of the discontinuity of energy, which acts as a medium of exchange.

2) It will be noticed that energy and space-time function in concert:

The energy-time coupling is dualistic. While energy seeks to become concentrated, time, quite to the contrary, tends to disperse. It is an eternal fratricidal struggle.
There is linear momentum. A body moves spatially due to the effect of a pulse. If this pulse stops, the body will continue moving in a straight line at a uniform speed. This is inertial movement.
If the body spins, or rotates around an axis, there are two directions of rotation that also develop energy.

You will find these three forms of deployment of energy in the time-space continuum in indeterminacy relations.

It should be noted that although, in classical mechanics, the magnitudes relating to energy and time-space are dealt with separately, they are inseparably linked in quantum terms. Here we are talking about the realm of AND whereas OR dominates in the classical field.

OR requires space-time to carry out the alternative.

AND is immediacy.

The combination of energy and space-time in quantum terms is not only irreversible but can only be spontaneous. They occur inversely to each other.